Copy link
Measures of Dispersion
Last updated: 07/07/2025
Key Points
- Measures of dispersion quantify the spread of data points, which provides context to datasets that measures of central tendency fail to capture.
- Common measurements of dispersion include range, interquartile range (IQR), variance, standard deviation (SD), and coefficient of variation (CV), each offering distinct insights depending on the purpose of analysis.
- Standard deviation and coefficient of variation are particularly useful in research, as they provide interpretability and a means to compare variability across different variables or measurement units.
Introduction
- Measures of central tendency (mean, median, mode) describe where data points are centered but do not indicate how spread out the data is around the center.1
- To capture variation or spread, measures of dispersion are used.1
- Commonly used measures of dispersion include:
- Range – the difference between the largest and smallest values1,2
- IQR – the spread of the middle 50% of values1,2
- SD – average distance of values from the mean2,3
- Variance (s²) – the squared average deviation from the mean4
- CV – standard deviation relative to the mean5
- These measures help describe the consistency or variability within a dataset.1
- Low variability indicates that values are closely grouped, reflecting higher precision.1
- High variability indicates that values are spread out, reflecting lower precision and greater dispersion.1
Range and IQR
Range
- The simplest measure of variability is the range, which is the difference between the largest and smallest points in a dataset.1,2
- Advantages:
- Easy and quick to calculate.2
- Disadvantages:
- It is based solely on two values and disregards the rest of the data.2
- It is highly sensitive to outliers, which can skew findings.2
Example:
Suppose a study records systolic blood pressures during anesthetic induction for 10 patients, with the following findings: 90, 93, 97, 99, 101, 102, 104, 106, 108, 145 mmHg.
- The range of this data set is 145 – 90 = 55 mmHg.
- However, 145 mmHg may represent an outlier.
- This makes the range a less accurate reflection of the overall dataset, where most values fall between 90 and 108 mmHg.
- In this situation, a more robust measure such as the IQR may offer a clearer representation of the data’s variability.
IQR
- An alternative to the range is the IQR, which provides a more robust measure of variability.1,2,4
- Quartiles divide a dataset into four equal parts.4
- Other subdivisions include:4
- Tertiles (three groups)
- Quintiles (five groups)
- Deciles (ten groups)
- The IQR represents the range between the first quartile (Q1) and the third quartile (Q3), capturing the middle 50% of the data.4
- IQR is resistant to outliers, making it useful when the data are skewed or not symmetrically distributed, which contrasts with range.2
Example:
A study records postoperative pain scores for 10 patients following surgery with the following scores: 2, 3, 4, 4, 5, 5, 6, 7, 8, 10.
IQR Calculation:
- Q1 (25th percentile): Average of the 3rd and 4th values = (4 + 4) / 2 = 4
- Q3 (75th percentile): Average of the 8th and 9th values = (7 + 8) / 2 = 7.5
- IQR = Q3 – Q1 = 7.5 – 4 = 3.5
Interpretation:
- Although the range is 8 (10-2), the IQR of 3.5 shows that the middle 50% of patients had pain scores between 4 and 7.5.
- Reporting the IQR gives a more accurate picture of patient experiences than the full range, which overemphasizes extreme values.
Variance, SD, and CV
Variance
- The sample variance (denoted as s²) is the average of the squared differences from the mean.1,3,4 It is calculated using the following formula:1
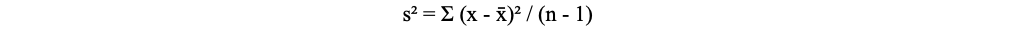
-
- x = each individual value
- x̄ = sample mean
- n = number of observations
- It provides a numerical estimate of data spread or dispersion.1
- It is always non-negative, as variance can never be less than zero.3
- Variance equals zero only when all values in the dataset are identical.3
- A smaller variance indicates that the values tend to congregate around the mean.3
- A larger variance suggests that at least some values are far from the mean.3
- Variance is the penultimate step in calculating standard deviation.1
Clinical Significance of Variance
- In clinical practice, variance helps determine how a patient’s measurement is compared to a reference population.3
- Reference ranges in laboratory tests (e.g., serum sodium or hematocrit) are typically calculated using the mean ± a multiple of the variance, helping clinicians interpret individual values.3
- In anesthesiology, understanding variance helps guide the selection of drugs.
- Medications with longer half-lives and slower metabolism often exhibit lower variability in blood levels, resulting in more consistent effects and a reduced risk of over- or underdosing.3
SD
- SD is the most widely used measure of dispersion in quantitative data.2
- It reflects how spread out the individual observations are around the mean.2,3
- Conceptually, SD answers “how far each value is from the mean?”6
- SD is calculated using the following formula2
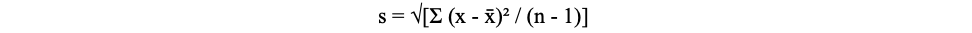
-
- x = each individual value
- x̄ = sample mean
- n = number of observations
- A small SD suggests that values congregate around the mean.1
- A large SD indicates that the data are widely spread out from the mean.6
SD and the Normal Distribution
- In a normally distributed dataset:2,6
- ~68.3% of values fall within the mean ± 1 SD
- ~95.5% fall within the mean ± 2 SD
- ~99.7% fall within the mean ± 3 SD
- This property allows SD to be used for probability estimates and standardized comparisons.6
Example:
A study measures the induction dose of propofol administered to 25 adult patients undergoing general anesthesia. The data shows the following:
- Mean dose = 2.0 mg/kg
- SD = 0.2 mg/kg
Interpretation:
- The mean indicates that, on average, patients received 2.0 mg/kg of propofol.
- The SD of 0.2 mg/kg suggests that most doses were relatively close to the mean, falling between 1.8 and 2.2 mg/kg.
- Assuming normal distribution, it can be expected that the following is true:
- ~68% of patients received between 1.8 and 2.2 mg/kg
- ~95% received between 1.6 and 2.4 mg/kg
CV
- The coefficient of variation (CV) is a measure of relative dispersion, expressing the standard deviation as a percentage of the mean.5
- It is calculated using the following formula:5
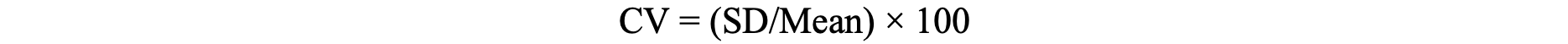
- CV allows for comparisons to be made between datasets that differ in scale or units.5
- The CV is unitless, making it especially useful when comparing variability across measurements that have different magnitudes (e.g., heart rate vs. blood pressure).5
- The CV is appropriate only when data are measured on a ratio scale which have a true zero point.5
- Standard methods for inference with CV often rely on the assumption that the data are normally distributed.5
References
- Mohan S, Su MK. Biostatistics and Epidemiology for the Toxicologist: Measures of Central Tendency and Variability-Where Is the "Middle?" and What Is the "Spread?". J Med Toxicol. 2022;18(3):235-8. Link
- Manikandan S. Measures of dispersion. J Pharmacol Pharmacother. 2011;2(4):315-6. Link
- Wadhwa RR, Azzam D. Variance. In: StatPearls. Treasure Island, FL: StatPearls Publishing; 2023. Accessed June 30, 2025. Link
- Whitley E, Ball J. Statistics review 1: presenting and summarising data. Crit Care. 2002;6(1):66-71. Link
- Botta-Dukát Z. Quartile coefficient of variation is more robust than CV for traits calculated as a ratio. Sci Rep. 2023;13(1):4671. Link
- Andrade C. Understanding the difference between standard deviation and standard error of the mean, and knowing when to use which. Indian J Psychol Med. 2020;42(4):409-10. Link
Copyright Information

This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.